| Table/Figure#
| Items
| EFD Data
| Submission Instruction
|
| Data file |
Image |
Image files |
Sample + Tecplot layout file |
| 1.7a-1 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 0.9625\) (SS 3/8)
Mean flow |
Refer to sample file for detail |
<Common>
Axis:
\(-0.035 < Y/L_{PP} < 0.035 \)
\(-0.065 < z/L_{PP} < 0.005 \)
Aspect ratio = 1:1
Frame line of SS 3/8 should be drawn.
<Contours>
Filename:
[Identifier]_S2-woDuct-wProp_U_1-7a.png (for \( u / U \) )
[Identifier]_S2-woDuct-wProp_V_1-7a.png (for \( v / U \) )
[Identifier]_S2-woDuct-wProp_W_1-7a.png (for \( w / U \) )
Contour style: lines
Line Style: solid positive, dashed negative
Contour intervals:
\( \Delta (u/U) = 0.1, \Delta (v/U) = 0.1, \Delta (w/U) = 0.1 \)
<Cross flow vectors>
Filename:
[Identifier]_S2-woDuct-wProp_VW_1-7a.png
Vector length: \(0.004\) [Magnitude/Grid Units]
Length of Reference vector: 1.0
|
Case1.7a_SPIV.zip |
| 1.7a-2-1 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 0.9843\)
(110[mm] ahead from AP)
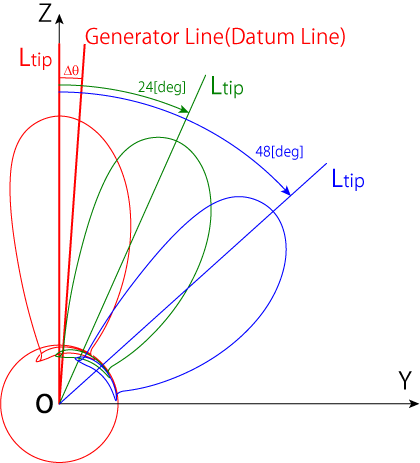
Blade angle is \(0\) [deg]
|
Refer to sample file for detail |
<Common>
Axis and Aspect ratio are same as Case1.7a-1
A propeller circle should be drawn.
<Contours>
Filename:
[Identifier]_S4-woDuct-wProp000_U_1-7a.png (for \( u / U \) )
[Identifier]_S4-woDuct-wProp000_V_1-7a.png (for \( v / U \) )
[Identifier]_S4-woDuct-wProp000_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S4-woDuct-wProp000_VW_1-7a.png
The others are same as Case1.7a-1
|
| 1.7a-2-2 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 0.9843\)
(between duct and propeller, 110[mm] ahead from AP)
Blade angle is \(24\) [deg]
|
Refer to sample file for detail |
<Common>
Same as Case1.7a-2-1
<Contours>
Filename:
[Identifier]_S4-woDuct-wProp024_U_1-7a.png (for \( u / U \) )
[Identifier]_S4-woDuct-wProp024_V_1-7a.png (for \( v / U \) )
[Identifier]_S4-woDuct-wProp024_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S4-woDuct-wProp024_VW_1-7a.png
The others are same as Case1.7a-1
|
| 1.7a-2-3 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 0.9843\)
(between duct and propeller, 110[mm] ahead from AP)
Blade angle is \(48\) [deg]
|
Refer to sample file for detail |
<Common>
Same as Case1.7a-2-1
<Contours>
Filename:
[Identifier]_S4-woDuct-wProp048_U_1-7a.png (for \( u / U \) )
[Identifier]_S4-woDuct-wProp048_V_1-7a.png (for \( v / U \) )
[Identifier]_S4-woDuct-wProp048_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S4-woDuct-wProp048_VW_1-7a.png
The others are same as Case1.7a-1
|
| 1.7a-3-1 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 1.0000\) (AP)
Blade angle is \(0\) [deg]
|
Refer to sample file for detail |
<Common>
Axis and Aspect ratio are same as Case1.7a-1
<Contours>
Filename:
[Identifier]_S7-woDuct-wProp000_U_1-7a.png (for \( u / U \) )
[Identifier]_S7-woDuct-wProp000_V_1-7a.png (for \( v / U \) )
[Identifier]_S7-woDuct-wProp000_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S7-woDuct-wProp000_VW_1-7a.png
The others are same as Case1.7a-1
|
| 1.7a-3-2 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 1.0000\) (AP)
Blade angle is \(24\) [deg]
|
Refer to sample file for detail |
<Common>
Same as Case1.7a-3-1
<Contours>
Filename:
[Identifier]_S7-woDuct-wProp024_U_1-7a.png (for \( u / U \) )
[Identifier]_S7-woDuct-wProp024_V_1-7a.png (for \( v / U \) )
[Identifier]_S7-woDuct-wProp024_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S7-woDuct-wProp024_VW_1-7a.png
The others are same as Case1.7a-1
|
| 1.7a-3-3 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 1.0000\) (AP)
Blade angle is \(48\) [deg]
|
Refer to sample file for detail |
<Common>
Same as Case1.7a-3-1
<Contours>
Filename:
[Identifier]_S7-woDuct-wProp048_U_1-7a.png (for \( u / U \) )
[Identifier]_S7-woDuct-wProp048_V_1-7a.png (for \( v / U \) )
[Identifier]_S7-woDuct-wProp048_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S7-woDuct-wProp048_VW_1-7a.png
The others are same as Case1.7a-1
|
| 1.7a-3-4 |
\(u, v, w\) contours and cross flow vectors at \(x/L_{PP} = 1.0000\) (AP)
Mean flow
|
Refer to sample file for detail |
<Common>
Same as Case1.7a-3-1
<Contours>
Filename:
[Identifier]_S7-woDuct-wPropmean_U_1-7a.png (for \( u / U \) )
[Identifier]_S7-woDuct-wPropmean_V_1-7a.png (for \( v / U \) )
[Identifier]_S7-woDuct-wPropmean_W_1-7a.png (for \( w / U \) )
The others are same as Case1.7a-1
<Cross flow vectors>
Filename:
[Identifier]_S7-woDuct-wPropmean_VW_1-7a.png
The others are same as Case1.7a-1
|